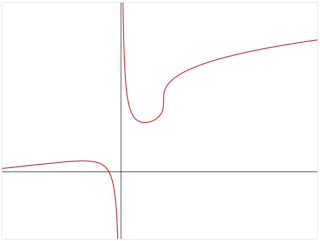
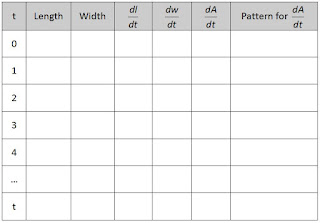
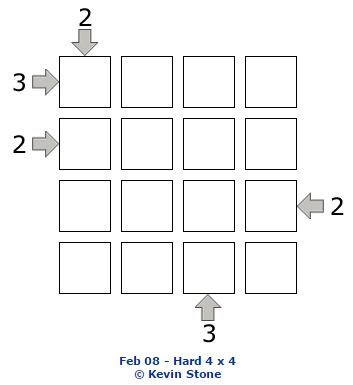
I was fortunate enough to be able to attend a half-day workshop on Thursday with Peter Liljedahl. I first heard about Peter's work a few years ago after he had spoken at a conference in Ottawa. There was much buzz from those who attended about VNPS and VRG, most notably from Alex Overwijk. Al was happy (!) to share all he had learned about vertical non-permanent surfaces and visible random groups (he may or may not have stopped strangers on the street to tell them about it). He has become Peter's #1 fan, even entitling the Ignite session he did last year "Things Peter Says". Although I had heard the vast majority of what Peter shared with us, there were a couple of important puzzle pieces that got filled in which make me believe that I can create what he refers to as a thinking classroom. This post is not intended to explain it all to you - for that you should visit Peter's website here or read this post from Alex.
I have used VNPS in my classroom for a few years now, but not every day and not instead of teaching/facilitating lessons. I use them for review stations and for tasks. I do visible random groups whenever I do group work, so that's not new for me (best group size = 3). I also spiral some of my courses with lots of activities, so I think that in many respects I already have a thinking classroom. My students tell me that even when I teach the same lesson as other teachers, I do it differently - I make them do the math, I don't just give it to them. But one can always do better... What convinced me was hearing about an actual curricular example of how to structure VNPS. Peter only spoke about students learning how to factor for a couple of minutes, but with enough detail to make it all click for me. I will attempt to share how I see it all working, but apologize in advance if my thoughts have still not gelled.
VNPS is a means to a goal, not the goal itself. It is, according to Peter's research, the most effective vehicle to creating a thinking classroom. One where all students are engaged in meaningful mathematics - doing the math, not watching someone else do it. They are learning to because autonomous, to look for the next question and persevere when they get stuck. The teacher's role in this is hard to nail down - you need to adjust to what you see constantly. It's structured chaos at its best. And I don't think it can be successful without really good planning (and I would highly recommend the book "5 Practices for Orchestrating Productive Mathematics Discussions" to help). Thursday's experience helped me see how all the pieces fit.
The structure looks like this:
- Start with a quick (~2 minute) lesson or prompt to activate prior learning or give students enough to build upon. Instructions should be oral as much as possible. If you give instructions in writing students have to decode them individually, whereas if they are oral instructions, students immediately start talking to each other.
- The questions they are working on must be sequenced in a logical way to develop the skills while keeping all students in "flow". These don't have to be incredible task questions - they can be everyday textbook questions. The importance of proper selection and sequencing is huge!
- There must be a lesson close that will level the class to the bottom. This could be a full-class debrief (going through a different example) or a gallery walk that is also thoughtfully sequenced.
I spend part of Friday with Sheri Walker working on sequencing questions for calculus (she was gracious enough to work on topics that she has already covered). Although not finalized, I think it helped us both think through how to make this work.
I still have lingering questions/concerns.
I worry - maybe that's too strong of a word - about the introverts in my classes. Especially the shy introverts. Because I am one, and I know how exhausting working in groups where you may not be entirely comfortable with the material or people can be. I am already purposeful about making my classroom a safe space for learning, which includes making mistakes, but I still worry...
I wonder about the number of markers and who is using them. We worked through two problems on Thursday and each group only received one marker. There were no rules around who should do the writing, but Peter was going around the room taking the marker from some and handing it to others. I was very aware of how long I had the marker when I was in my first group and did my best to always put the marker down when I had finished with it. It is much easier to pick a marker up than to take it out of someone's hand. There were questions about all of this and suggestions of either using a timer so that each person had the marker for an equal-ish amount of time or that the person with the marker could only write others' ideas. This takes me back to the introverts issue - I would hate to be the one who had to write someone else's ideas if I didn't understand them. But I also know that I did not touch the marker in my second group, so it's not that hard to step back a little which is not what we want.
I don't know if I can make this work with my grade 10 applied class. MFM2P is generally made up of students where one half to two thirds have IEPs. Many require written instructions. Many cannot work in groups, only pairs. Many cannot work with certain other students in the class. Many (most?) hate math and are often very unwilling to do any work. There seem to be so many obstacles with that group, that I'm not certain this is the way to go. Spiralling with activities has really helped with engagement and success, so I think the VNPS may continue to be an every-so-often thing. If you can convince me otherwise, I would love to hear your thoughts!
Peter says we shouldn't give students notes. I agree, however, I will still continue to post notes on Google Classroom in calculus because there would be a mutiny if I didn't. There are only so many battles that I will take on! I like the idea of finishing the "lesson" at around the 50-60 minute mark, doing the lesson close then leaving ~15 minutes for students to write down, in their own words, what they have learned. They will then be able to reference my posted lesson with examples as they need.
There is so much more to all of this, but this is where I am for now. I am not promising to blog every day, but I will write about how it's all going. I would love to hear your thoughts in the comments. Thanks.