I am not doing a daily blog about my grade 10 applied class this semester. This is not because everything is the same as last year or eve last semester. In fact, I have changed almost everything so far in this first cycle. I have different warm-ups, am doing topics in a different order and have made some new resources, too. If I'm not happy with things, I cannot leave them alone. I have thought about sharing what I have done at the end of each cycle - if that's of interest to you, please let me know.
Last year during a lesson study process we looked at an activity that involved students creating towers out of linking cubes and competing to see who could get the tallest tower. This is what I mean by linking cubes (also known as cube-a-links and unifix cubes):
I believe the students all had cards that told them how many cubes to begin with and how many to add each time. It went fairly well, but once a student was "out" (because their tower fell), they were no longer really engaged. Anyway, that is what inspired today's activity which will be my students first look at solving systems of linear equations. I am trying to have them really understand how the starting value and rate of change will affect their towers (and corresponding graphs). We have done a few visual patterns and solved some equations with a variable on both sides, so I think they will be ready for this.
Here is the file. I would love feedback. I will add a postscript if it's a disaster ;)
Wednesday 24 February 2016
Monday 22 February 2016
The Power of Popsicle Sticks
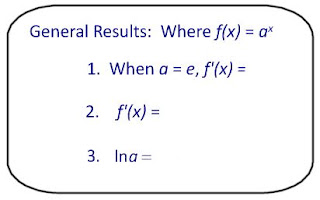
Content is not important to this post, but it sets the context. We looked at derivatives of exponential functions on Friday, starting with the derivative of y = ex. We do this by looking at values of f(x) and f'(x) and then calculating the ratio of f'(x) to f(x). Today, we explored finding derivatives of exponential functions from first principles. I therefore didn't think there would be any issues when I asked them all to complete this:
And I might not have known that this was neither clear nor obvious had it not been for Popsicle sticks.
This is my tin of Popsicle sticks for my morning class. I have one for each of my afternoon classes as well. I generally go with the "no hands up except to ask a question" rule which means that the one or two extroverts in the class who have all the answers are not the only voices heard. I choose a name randomly to answer a question and then quite often choose another name to add thoughts to the first response. A response of "I don't know" is okay and is often followed by several other "I don't know"s which tells me that we all need to take a step back.
This morning's Popsicle sticks told me a lot. I went through a lot of sticks - there was a huge pile outside the tin - which means that there were many answers (some right, some not) to my questions and much "What do you think?" from me as I chose another name following each answer. When this happen I have them talk in their groups to see if they can make connections together before trying again.
The Popsicle sticks help make my classroom a learning space where everyone has a voice and every voice is important. I do my utmost to make it a safe place where making mistakes is not only okay, but important. In addition to that, Popsicle sticks help me be a better teacher. They help me gauge the understanding in the room (I do thumbs up-sideways-down a lot, also) and help adjust the pace and choose what we need to practice in the moment. This is still a work in progress for me, but one that I think is important to help me grow as a teacher and to ensure that my students truly understand what we are doing, not merely mimic completed examples.
I first read about using Popsicle sticks in Dylan Wiliam's Embedded Formative Assessment. Here is a video about this strategy and here is his website.
And I might not have known that this was neither clear nor obvious had it not been for Popsicle sticks.
This is my tin of Popsicle sticks for my morning class. I have one for each of my afternoon classes as well. I generally go with the "no hands up except to ask a question" rule which means that the one or two extroverts in the class who have all the answers are not the only voices heard. I choose a name randomly to answer a question and then quite often choose another name to add thoughts to the first response. A response of "I don't know" is okay and is often followed by several other "I don't know"s which tells me that we all need to take a step back.
This morning's Popsicle sticks told me a lot. I went through a lot of sticks - there was a huge pile outside the tin - which means that there were many answers (some right, some not) to my questions and much "What do you think?" from me as I chose another name following each answer. When this happen I have them talk in their groups to see if they can make connections together before trying again.
The Popsicle sticks help make my classroom a learning space where everyone has a voice and every voice is important. I do my utmost to make it a safe place where making mistakes is not only okay, but important. In addition to that, Popsicle sticks help me be a better teacher. They help me gauge the understanding in the room (I do thumbs up-sideways-down a lot, also) and help adjust the pace and choose what we need to practice in the moment. This is still a work in progress for me, but one that I think is important to help me grow as a teacher and to ensure that my students truly understand what we are doing, not merely mimic completed examples.
I first read about using Popsicle sticks in Dylan Wiliam's Embedded Formative Assessment. Here is a video about this strategy and here is his website.
Saturday 20 February 2016
Log Clothesline - My Post-Activity Post
Yesterday, I ran the log clothesline activity with two of my classes.
What I liked:
What I would change:
What I liked:
- The great math conversations. They had not worked with logs for a while now, so some remembered a lot and some, well, not so much. They were talking to each other about how to get going with tricky expressions at their desks initially and the conversations continued when they were at the clothesline trying to place the cards in the correct order.
- The collaboration. Those who couldn't even remember how to go from logarithmic form to exponential form got help in their groups. They asked each other questions, they checked each other's work and argued about who was right.
- The struggles. Apart from the one card with a typo (oops!), all of the others worked out. However, I made this a no calculator activity (horror!) so they had to work smarter to reduce the amount of arithmetic required. Those log rules really became useful.
What I would change:
- I found this activity worked much more smoothly with my smaller afternoon class. I only had 13 students in that class yesterday and everyone was engaged and busy. My morning class, which had 24 students, had more "traffic jams" around the clothesline causing other students to step away and no longer be engaged in the activity. I would put up two clotheslines for a larger class next time.
- I also liked having extra cards in my afternoon class for those who finished quickly, so I would make more cards next time or have two sets for a larger class.
- The change that would have the biggest impact, I believe, is adding more number markers to the line. I had a 0 marker, but the struggle to correctly arrange all the expressions on the line was greater than I had planned. I asked my afternoon class if they thought having more number markers would help, and they unanimously said yes! I loved that some were using the small whiteboards to work out the expressions from other students' cards that were already on the line, but it became overwhelming.
I definitely think this activity was worth doing and that all students got something out of it. Here is the final clothesline:
Wednesday 17 February 2016
Log Clothesline
There has been quite the buzz around clothesline activities of late. There is even a clotheslinemath.com website! I first heard about it from Andrew Stadel when he wrote this post. More recently, Jon Orr made a cool one to practice finding slope. Here is a link to his blog post. I thought that it would be great to use a clothesline to practice evaluating logs, but was having trouble finding the time to actually create it. Then we got a snow day (school buses are cancelled, but teachers still have to report to work... all of the students at my school take the bus so we have a day with no students) but I had other things to do (like meetings) and we were allowed to go home early (we got 51.2 cm of snow by the end of the day!). And then, shockingly, they cancelled the buses again today! So here is the log clothesline for you. I plan on actually doing it with my two MCV4U classes on Friday and and will snap some pictures then. I did take a "fake" one of a colleague placing a card on the clothesline.
Here is the Word file, and the PDF version is here. And I'll even give you the answers. I numbered the pages so those correspond to the question numbers, which I have sorted in the Excel file so that I can quickly check for correctness. I printed two pages per sheet and cut them down the middle.
I will give out one card to each student and they will have to evaluate their expression and check at least one from someone else in their group. Once the whole group is confident in their answers, they will go to the clothesline to place their expression in the correct location. I will give them a 0 marker on the number (clothes)line. The trick is that I will tell them that they can't write on the cards so in order to figure out where their card goes, they will have to work out a number of other expressions. Those students who put their cards up first will be responsible for ensuring that all the cards that follow are in the right place.
I will try to write a "how it went" blog post after I run the activity.
Postscript
After hitting publish this morning I started to think more about this activity and started questioning whether it met the spirit of clothesline activities. I worried that as it doesn't really work students' number sense, it might not be an activity worthy of sharing. I sought advice and, though we agreed this would be better suited to an intro to logs activity (more on that in a minute), this practice, with built-in error analysis and collaboration, was worth sharing.
Back to the intro to logs idea. This is what I envision: students get cards with powers of 2s from 1/64 to 32768 (or something like that, depending on the number of students in the class) and they have to attempt to place them on a clothesline that will have markers of 0 and 1 on it (not as shown below). It would look something like this:
Hopefully it will become clear that there are too many cards between 0 and 1 and that the larger numbers simply cannot fit on the clothesline. So what to do? I'm not sure how to introduce the idea of taking log base 2 of each of the numbers, but that will be the goal. The result will be a clothesline with a logarithmic scale which will allow all the numbers to be seen. I won't actually be able to do this until next fall, so please let me know if you try it out!
Here is the Word file, and the PDF version is here. And I'll even give you the answers. I numbered the pages so those correspond to the question numbers, which I have sorted in the Excel file so that I can quickly check for correctness. I printed two pages per sheet and cut them down the middle.
I will give out one card to each student and they will have to evaluate their expression and check at least one from someone else in their group. Once the whole group is confident in their answers, they will go to the clothesline to place their expression in the correct location. I will give them a 0 marker on the number (clothes)line. The trick is that I will tell them that they can't write on the cards so in order to figure out where their card goes, they will have to work out a number of other expressions. Those students who put their cards up first will be responsible for ensuring that all the cards that follow are in the right place.
I will try to write a "how it went" blog post after I run the activity.
Postscript
After hitting publish this morning I started to think more about this activity and started questioning whether it met the spirit of clothesline activities. I worried that as it doesn't really work students' number sense, it might not be an activity worthy of sharing. I sought advice and, though we agreed this would be better suited to an intro to logs activity (more on that in a minute), this practice, with built-in error analysis and collaboration, was worth sharing.
Back to the intro to logs idea. This is what I envision: students get cards with powers of 2s from 1/64 to 32768 (or something like that, depending on the number of students in the class) and they have to attempt to place them on a clothesline that will have markers of 0 and 1 on it (not as shown below). It would look something like this:
Hopefully it will become clear that there are too many cards between 0 and 1 and that the larger numbers simply cannot fit on the clothesline. So what to do? I'm not sure how to introduce the idea of taking log base 2 of each of the numbers, but that will be the goal. The result will be a clothesline with a logarithmic scale which will allow all the numbers to be seen. I won't actually be able to do this until next fall, so please let me know if you try it out!
Thursday 4 February 2016
Small Changes
I have taught this weird grade 12 course we have in Ontario called Calculus & Vectors forever (well, since it was introduced) so I could just walk in each day and teach based on what I did last year. There are certainly days when that is exactly what will happen, but I am trying to tweak lessons as I go through. I have a little more time to do this as I am not revamping an entire course the way I did last semester.
Day 1: I really liked the Desmos activity I created last year so I turned it into an Activity Builder activity - here is the link. I loved being able to see what equations my students were trying so that I could give meaningful feedback if they were on the wrong path. There was a lot of good mathematical talk going on as well as some struggle which some students are clearly not used to. Many expected me to just tell them the answer when they got stuck. I did not.
Day 2: School buses were cancelled due to wicked freezing rain (the roads literally had a sheet of ice on them). Our schools never close so I had to report, but as all of our students take the bus in, I had a day without students.
Day 3: Limits. We don't spend a lot of time on limits, but I think there is value in understanding what a limit is as we head toward derivatives. In the past I have done a demonstration simulating the amount of medicine in your body using a pitcher of water and some food colouring. I have also talked about Archimedes, but switched things up this year. I let my students be Archimedes (only with calculators!) and paired them up to find the area of a regular polygon. As a class they chose a radius and we talked briefly about "apothem", a word they had never come across before.
This is what we ended up with:
Those blanks are groups that just didn't get there. This wasn't as obvious as you might think as they haven't done any work with polygons since grade 9. The area for the nonagon was erased when the group saw that an area of around 110 was not following the trend. I took a little time to ask them how they had found the area and was pleased at the number of different approaches they used to find the side length of the polygon. When I asked what they noticed they said the numbers were increasing. I asked how they were increasing and then what they might be approaching. One student guessed 80 or maybe 78. Another went straight to the area of a circle. (I don't yet know who not to call upon!)
Then we talked about Archimedes before defining a limit.
At this point they had a rough idea of what a limit was and a definition, but that doesn't necessarily translate into being able to find a limit. In past years I have given them the graph below along with 12 limits for them to find. It seemed to go well, but there were always some students who clearly had not understood any of it. So I changed my approach and projected the graph for them and asked them to write the value of the limit I said aloud on their little whiteboard. Just the number. Write it down then everyone holds up their whiteboards facing me so that I can see how they are doing. It was so good! I first asked for the limit as x approached -6 from the left. The class was pretty much split between -2 and 2.5, with a few not wanting to write anything down. I didn't say anything about their answers, instead I moved on and asked them the limit as x approached -6 from the right. The class unanimously wrote 2.5. Then we talked about both of these limits before I asked for the limit as x approached -6. Hmmm. Some wrote both previous answers, some wrote only one of them, some averaged them, some said IDK. This provided the opportunity for good discussion and reflection. We moved on to looking at the limit as x approached 5. There was even a "what if we..." question that segued into looking at the limit as x approached -1. Yes!
Then I handed out the same graph with those 12 limits we have always found and they did them without hesitation. We answered questions together and they really seemed to get it.
Next, we looked at limits of various functions given their equations. Everything was tied back to the graphs. We visualized what the graph was doing as we approached whatever value. I probably asked "Is there anything weird going on?" too many times, but I think it helps them think about whether the is a discontinuity and whether that impacts the limit.
As you have gotten this far, thanks for reading my blog. I was pumped coming out of that class and wanted to blog about it. It is strange to not blog every day!
Day 1: I really liked the Desmos activity I created last year so I turned it into an Activity Builder activity - here is the link. I loved being able to see what equations my students were trying so that I could give meaningful feedback if they were on the wrong path. There was a lot of good mathematical talk going on as well as some struggle which some students are clearly not used to. Many expected me to just tell them the answer when they got stuck. I did not.
Day 2: School buses were cancelled due to wicked freezing rain (the roads literally had a sheet of ice on them). Our schools never close so I had to report, but as all of our students take the bus in, I had a day without students.
Day 3: Limits. We don't spend a lot of time on limits, but I think there is value in understanding what a limit is as we head toward derivatives. In the past I have done a demonstration simulating the amount of medicine in your body using a pitcher of water and some food colouring. I have also talked about Archimedes, but switched things up this year. I let my students be Archimedes (only with calculators!) and paired them up to find the area of a regular polygon. As a class they chose a radius and we talked briefly about "apothem", a word they had never come across before.
This is what we ended up with:
Those blanks are groups that just didn't get there. This wasn't as obvious as you might think as they haven't done any work with polygons since grade 9. The area for the nonagon was erased when the group saw that an area of around 110 was not following the trend. I took a little time to ask them how they had found the area and was pleased at the number of different approaches they used to find the side length of the polygon. When I asked what they noticed they said the numbers were increasing. I asked how they were increasing and then what they might be approaching. One student guessed 80 or maybe 78. Another went straight to the area of a circle. (I don't yet know who not to call upon!)
Then we talked about Archimedes before defining a limit.
At this point they had a rough idea of what a limit was and a definition, but that doesn't necessarily translate into being able to find a limit. In past years I have given them the graph below along with 12 limits for them to find. It seemed to go well, but there were always some students who clearly had not understood any of it. So I changed my approach and projected the graph for them and asked them to write the value of the limit I said aloud on their little whiteboard. Just the number. Write it down then everyone holds up their whiteboards facing me so that I can see how they are doing. It was so good! I first asked for the limit as x approached -6 from the left. The class was pretty much split between -2 and 2.5, with a few not wanting to write anything down. I didn't say anything about their answers, instead I moved on and asked them the limit as x approached -6 from the right. The class unanimously wrote 2.5. Then we talked about both of these limits before I asked for the limit as x approached -6. Hmmm. Some wrote both previous answers, some wrote only one of them, some averaged them, some said IDK. This provided the opportunity for good discussion and reflection. We moved on to looking at the limit as x approached 5. There was even a "what if we..." question that segued into looking at the limit as x approached -1. Yes!
Then I handed out the same graph with those 12 limits we have always found and they did them without hesitation. We answered questions together and they really seemed to get it.
Next, we looked at limits of various functions given their equations. Everything was tied back to the graphs. We visualized what the graph was doing as we approached whatever value. I probably asked "Is there anything weird going on?" too many times, but I think it helps them think about whether the is a discontinuity and whether that impacts the limit.
As you have gotten this far, thanks for reading my blog. I was pumped coming out of that class and wanted to blog about it. It is strange to not blog every day!
Subscribe to:
Posts (Atom)